Sec x is the derivative of tan x. Let’s review some facts about tan x. Tan x in a right-angled triangle is the ratio of the opposite side of x to the adjacent side of x and thus it can be written as (sin x)/(cos x). This is used to differentiate between tan and x.
Let us learn the differentiation of tan x along with its proof in different methods and also we will solve a few examples using the derivative of tan x.
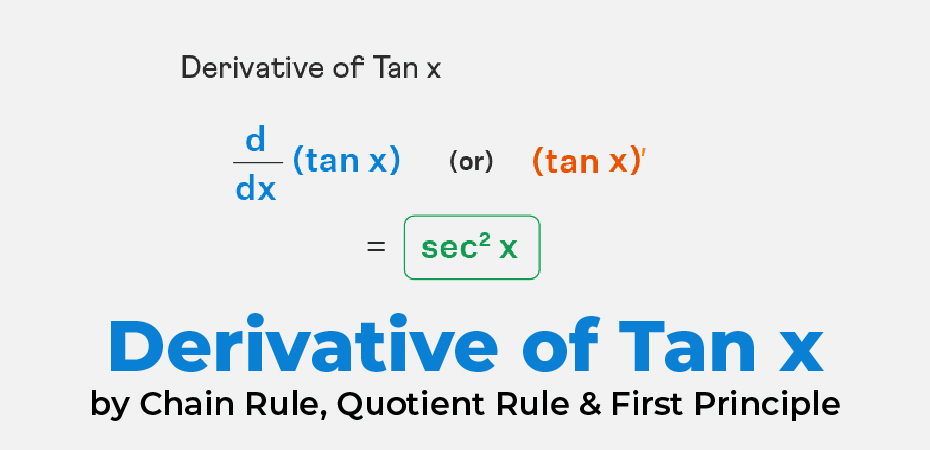
What is the Derivative of Tan x?
The derivative of tan x with respect to x is denoted by d/dx (tan x) (or) (tan x)’ and its value is equal to sec2x. Tan x is differentiable in its domain. To establish the differentiation of tan x to be sec2x, we use the existing trigonometric identities and existing rules of differentiation. We can prove this in the following methods:
- Proof by the first principle
- Proof by chain rule
- Proof by quotient rule
Derivative of Tan x Formula
The formula for differentiation of tan x is,
- d/dx (tan x) = sec2x (or)
- (tan x)’ = sec2x
Now we will prove this in different methods in the upcoming sections.

Derivative of Tan x Proof by First Principle
To find the derivative of tan x, we assume that f(x) = tan x. Then by first principle, its derivative is given by the following limit.
f'(x) = limₕ→₀ [f(x + h) – f(x)] / h … (1)
Since f(x) = tan x, we have f(x + h) = tan (x + h).
Substituting these in (1),
f'(x) = limₕ→₀ [tan(x + h) – tan x] / h
= limₕ→₀[ [sin (x + h) / cos (x + h)] – [sin x / cos x] ] / h
= limₕ→₀[ [sin (x + h ) cos x – cos (x + h) sin x] / [cos x · cos(x + h)] ]/ h
By sum and difference formulas, sin A cos B – cos A sin B = sin (A – B).
f'(x) = limₕ→₀ [ sin (x + h – x) ] / [ h cos x · cos(x + h)]
= limₕ→₀[ sin h ] / [ h cos x · cos(x + h)]
= limₕ→₀ (sin h)/ h ·limₕ→₀ 1 / [cos x · cos(x + h)]
By limit formulas, limₕ→₀ (sin h)/ h = 1.
f'(x) = 1 [ 1 / (cos x · cos(x + 0))] = 1/cos2x
We know that the reciprocal of cos is sec. So,
f'(x) = sec2x.
Hence proved.

Derivative of Tan x Proof by Chain Rule
We will prove the differentiation of tan x formula by chain rule. For this let us note that we can write y = tan x as y = 1 / (cot x) = (cot x)-1. Now, by power rule and chain rule,
y’ = (-1) (cot x)-2 · d/dx (cot x)
We have d/dx (cot x) = -csc2x. Also, by a property of exponents, a-m = 1/am.
y’ = -1/cot2x · (-csc2x)
y’ = tan2x · csc2x
Now, tan x = (sin x)/(cos x) and csc x = 1/(sin x). So
y’ = (sin2x)/(cos2x) · (1/sin2x)
= 1/cos2x
We have 1/cos x = sec x. So
y’ = sec2x
Hence proved.
Derivative of Tan x Proof by Quotient Rule
We can evaluate the quotient rule to derive the formula of the derivative of tan x. For this, we have to write tan x as a fraction. We know that tan x = (sin x)/(cos x). So we assume that y = (sin x)/(cos x). Then by quotient rule,
y’ = [ cos x · d/dx (sin x) – sin x · d/dx (cos x)] / (cos2x)
= [cos x · cos x – sin x (-sin x)] / (cos2x)
= [cos2x + sin2x] / (cos2x)
By one of the Pythagorean identities, cos2x + sin2x = 1. So
y’ = 1 / (cos2x) = sec2x
Hence proved. This proof is the most leisurely one among all the other proofs of the derivatives of tan x.
Common Misconceptions Related to Derivative of Tan x:
Here is some clarity concerning some common misconceptions regarding the differentiation of tan x.
- d/dx (tan x) is NOT equal to d/dx(sin x) / d/dx (cos x). Instead, we have to use the quotient rule to find the derivative of tan x (by writing it as (sin x)/(cos x)).
- d/dx (tan x) is NOT cot x. cot x is just the reciprocal of tan x.
- The derivatives of tan x and tan-1x are NOT same.
d/dx(tan x) = sec2x
d/dx(tan-1x) = 1/(1 + x2)
FAQs
Q: What is the Derivative of Tan x with Respect to x?
A; The derivative of tan x with respect to x is the square of sec x. i.e., d/dx(tan x) = sec2x. It can also be written as (tan x)’ = sec2x.
Q: What is the Derivative of Tan x2?
A: We know that d/dx(tan x) = sec2x. So d/dx(tan x2) = sec2x2 d/dx(x2) = 2xsec2x2 (by chain rule).
Q: What is the Differentiation of Tan x in Terms of Cos x?
A: We know that the derivative of tan x is sec2x. Also, sec x = 1/(cos x). So d/d(tan x) = 1/cos2x.
Q: What is the Derivative of tan x-1?
A: By using the derivative of tan x and chain rule, d/dx(tan x-1) = sec2x-1 d/dx(x-1) = sec2x-1 (-1 x-2) = (-sec2x-1)/(x2).
Q: Is the Derivative of Tan x Equal to Derivativative of Tan-1x?
A; No, the derivatives of tan x and tan-1x are different. The derivative of tan x is sec2x whereas the derivative of tan⁻¹x is 1/(1 + x2).
Q: What is the Difference Between the Derivative of Tan x and the Antiderivative of Tan x?
A: The derivative of tan x is sec2x. The antiderivative of tan x is nothing but the integral of tan x and ∫ tan x dx = ln |sec x| + C.